E-mail Alert
RSS
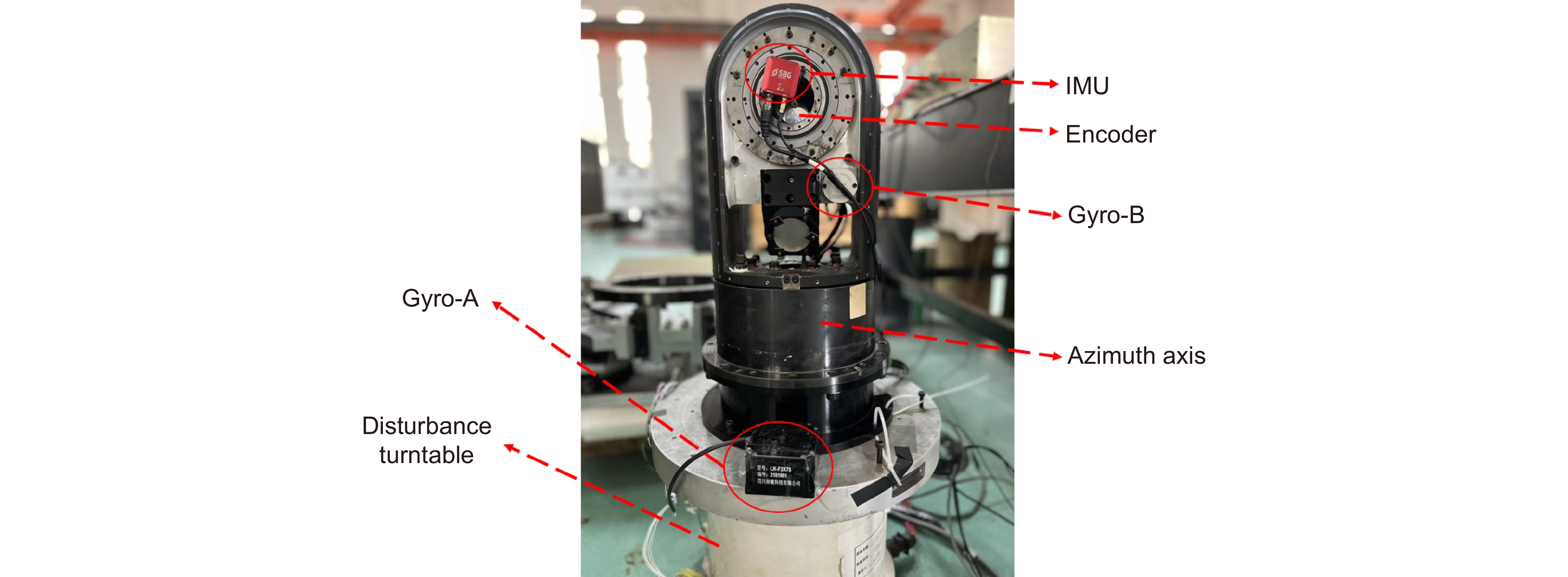
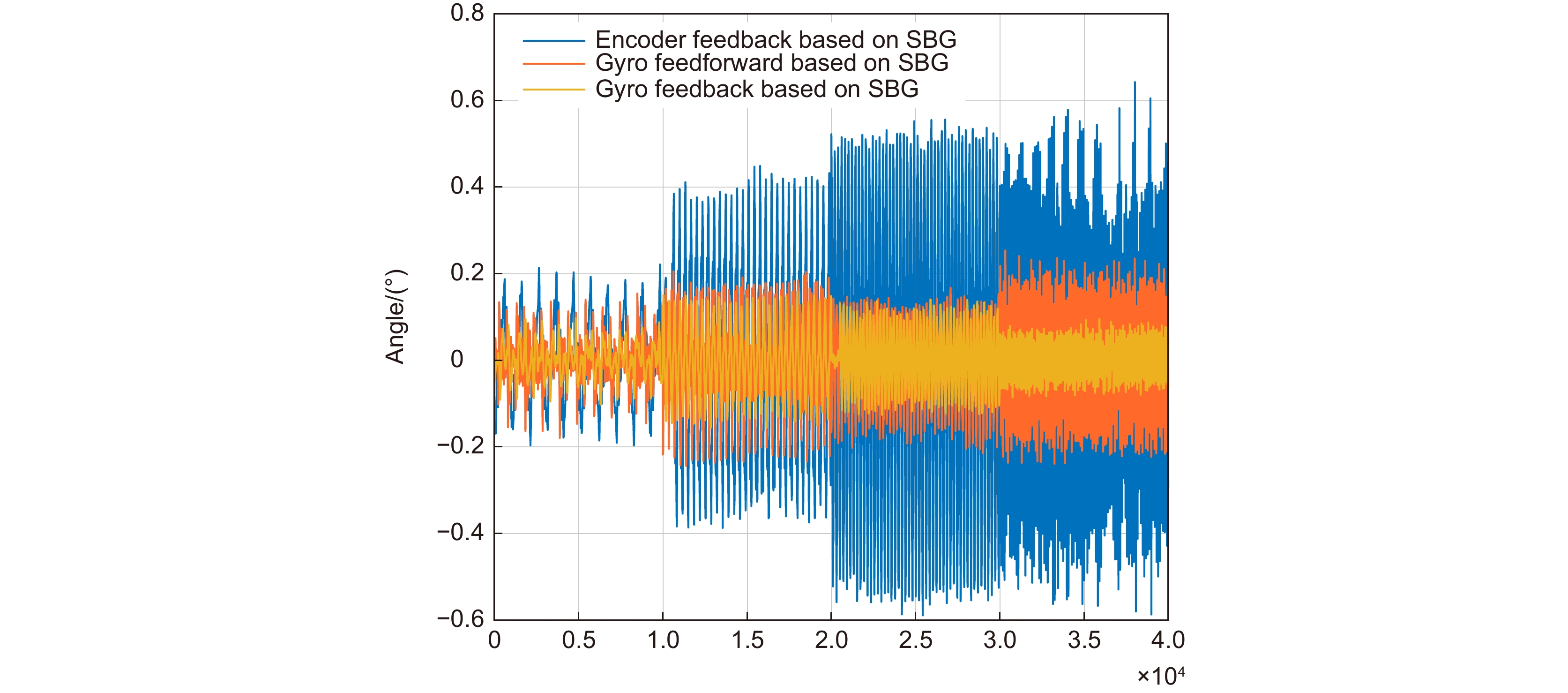
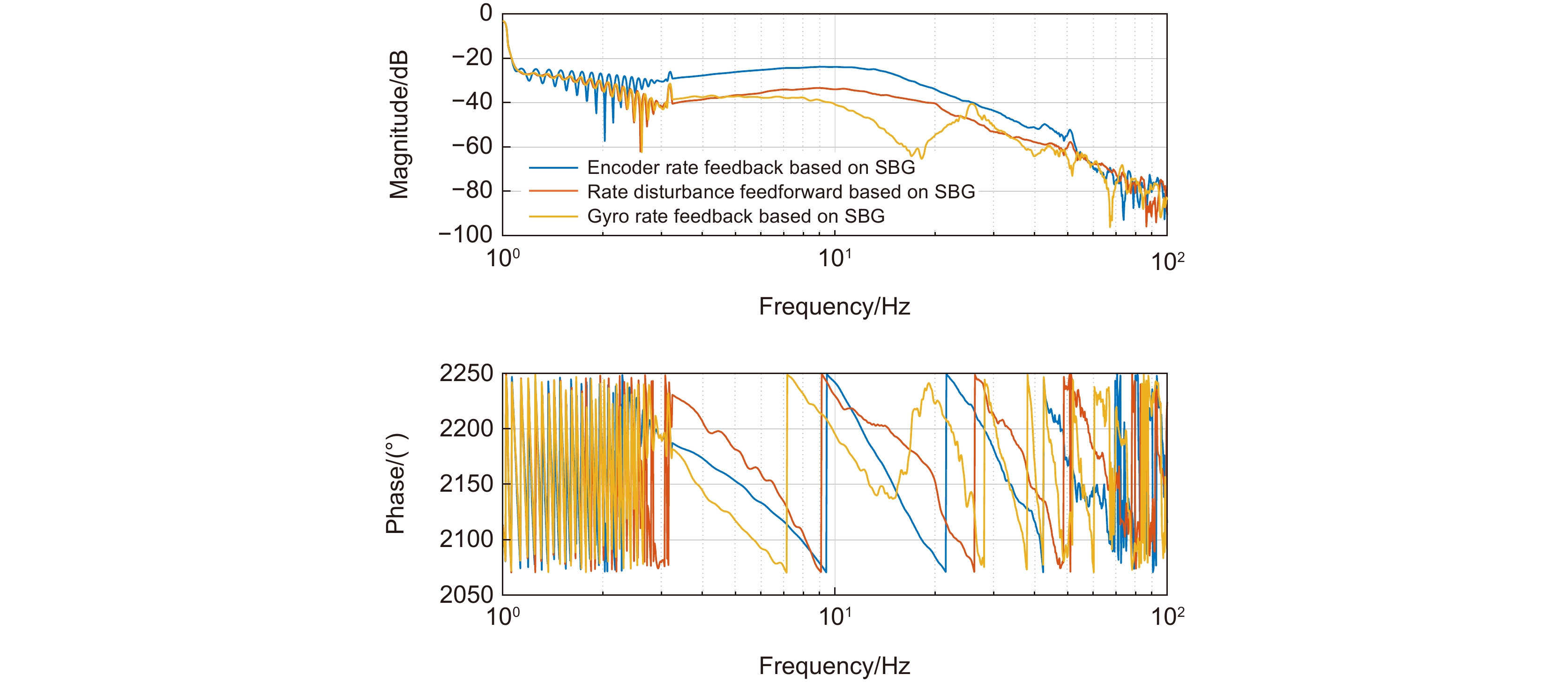
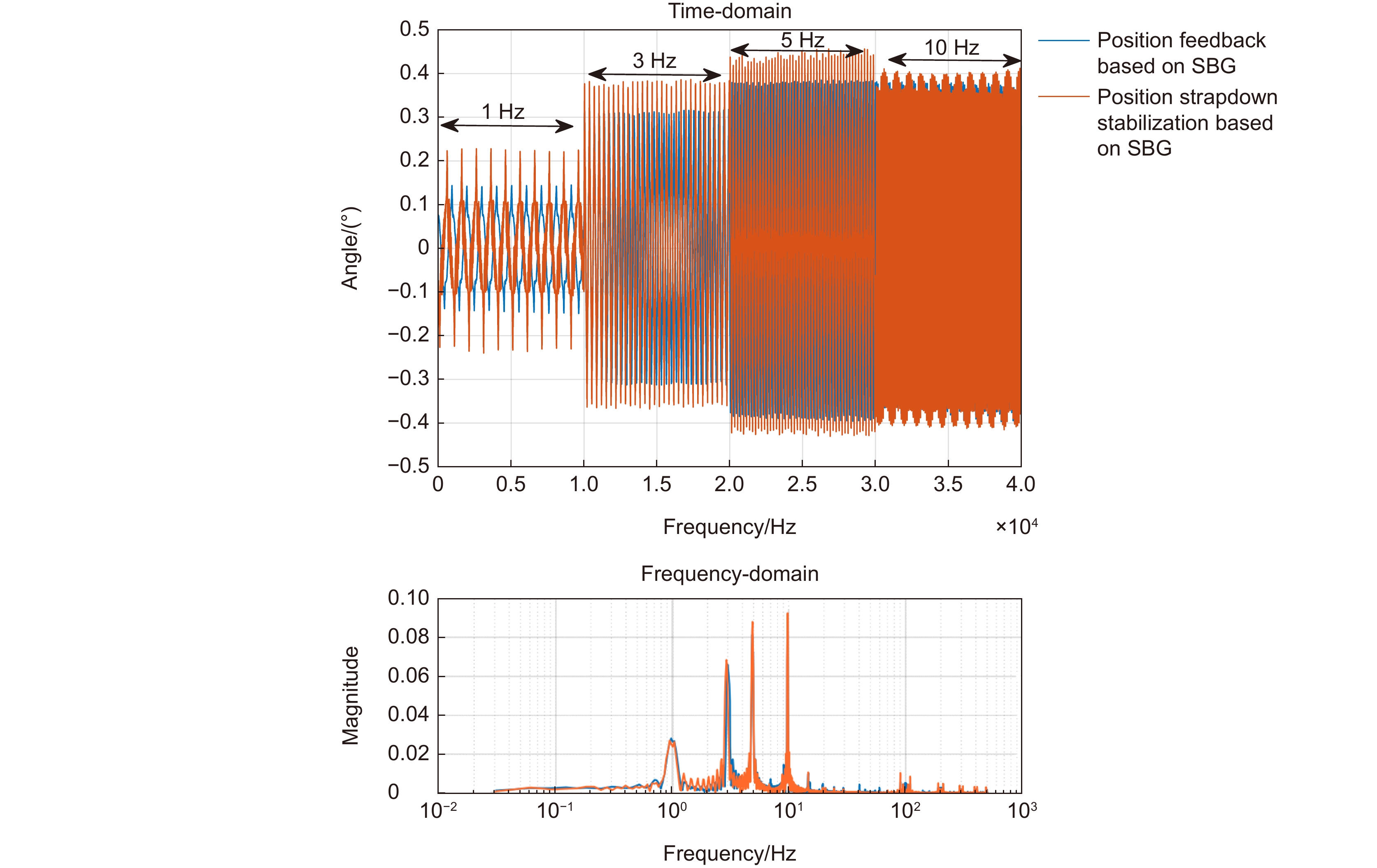
Strapdown inertial stabilization technology based on SBG inertial navigation in inertial stabilization gimbal
-
摘要:
轻小型SBG惯性导航系统具有体积小重量轻的特点，可以用于光电跟踪系统的直接及捷联位置稳定。本文研究了万向架中基于轻小型SBG惯导的五种惯性稳定控制方法，并进行了理论分析及实验验证。经典位置捷联稳定技术由于位置带宽的限制扰动抑制能力有限，难以满足高精度稳定要求。本文提出了一种基于SBG的位置速度双扰动前馈的稳定方法，并通过引入高通滤波器实现扰动前馈的解耦，可以进一步提升系统扰动抑制带宽以及稳定能力。理论分析和实验结果表明：在不考虑应用条件的限制时，虽然受到带宽的限制，基于SBG的陀螺直接稳定控制方法扰动抑制效果较好；而在平台体积重量受到限制的情况下，所提出的基于SBG的双扰动捷联稳定可以进一步提升系统扰动抑制能力，获得更好的稳定精度。
Abstract:The lightweight SBG inertial navigation system has the characteristics of being small and light, which can be used for the direct and strapdown stabilization of the electro-optical tracking system. In this paper, five inertial stabilization control methods based on the lightweight SBG navigation system in gimbals are studied, and theoretical analysis and experimental validation are performed. The classical position strapdown stabilization technique has limited disturbance suppression capability due to the limitation of position bandwidth, which makes it difficult to meet the requirement of high-precision stabilization. A SBG-based position-rate dual strapdown feedforward stabilization method is proposed, and the decoupling of the strapdown feedforward is achieved by introducing a high-pass filter, which can further improve the perturbation rejection bandwidth as well as the stabilization capability of the system. Theoretical analysis and experimental results show that the SBG-based gyro direct stabilization control method is better at disturbance suppression when the application conditions are not considered, although it is limited by the bandwidth. Under the limitations of platform volume and weight, the proposed SBG-based dual disturbance strapdown stabilization can further improve the system's disturbance rejection capability and obtain better stabilization accuracy.
-
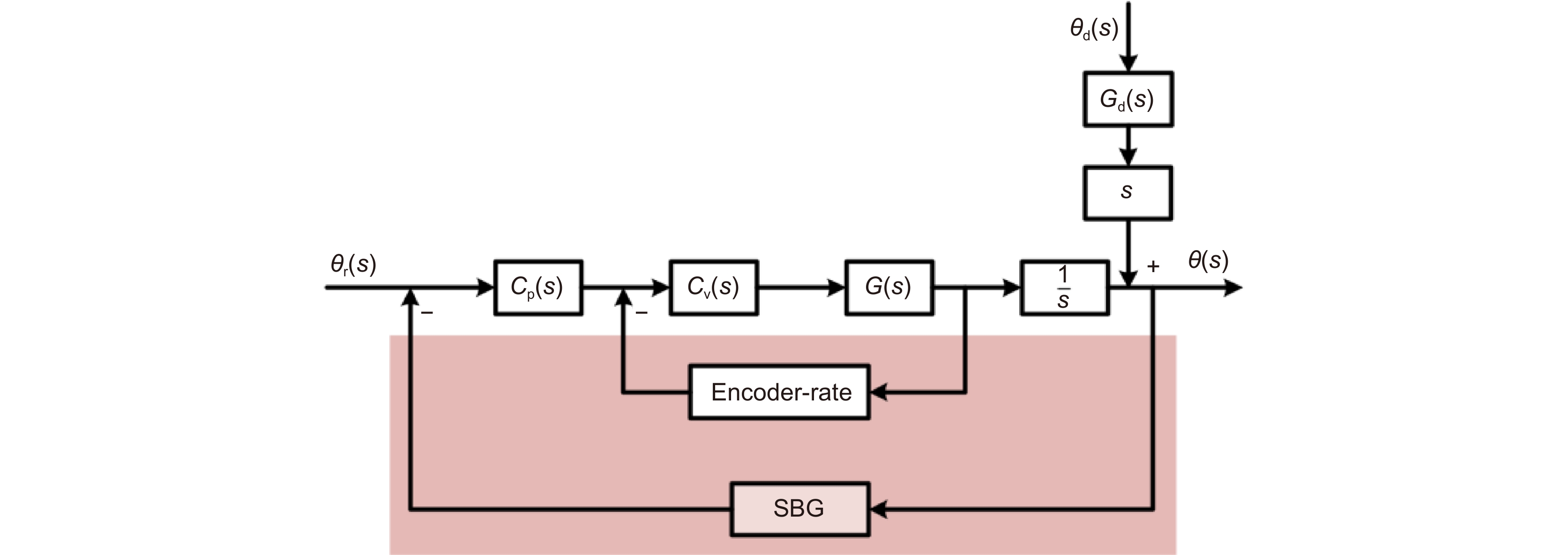
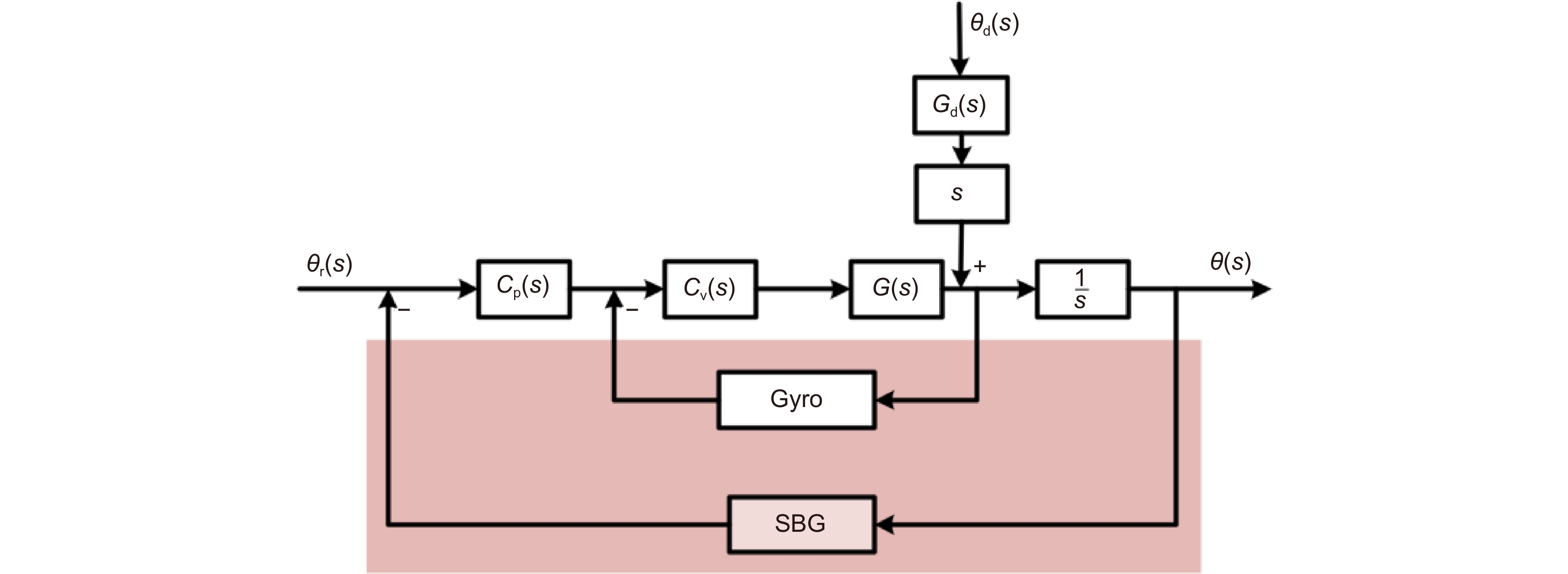
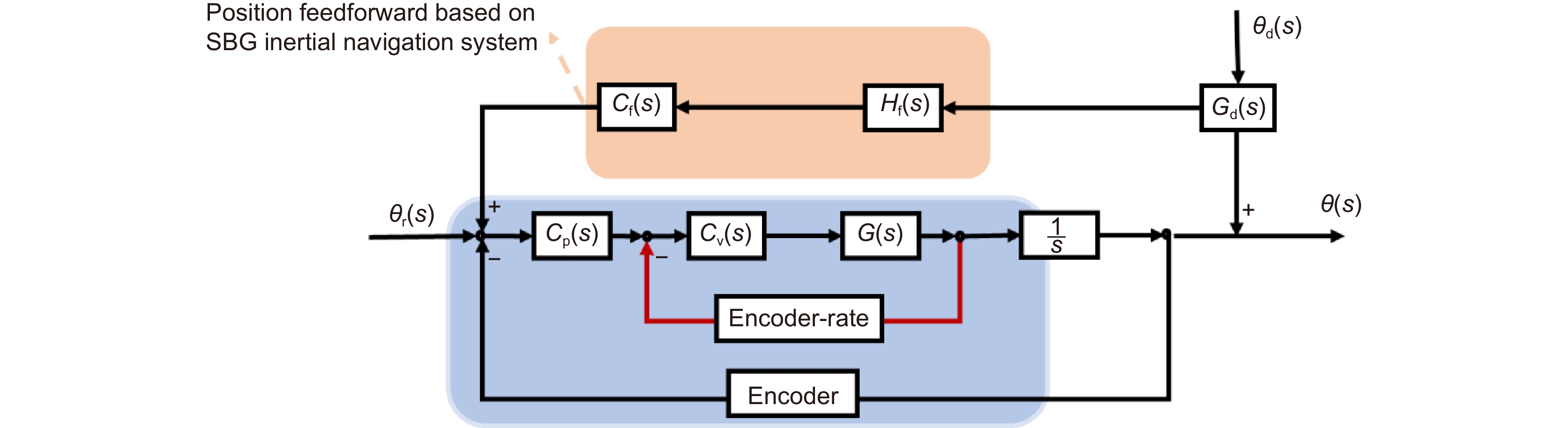
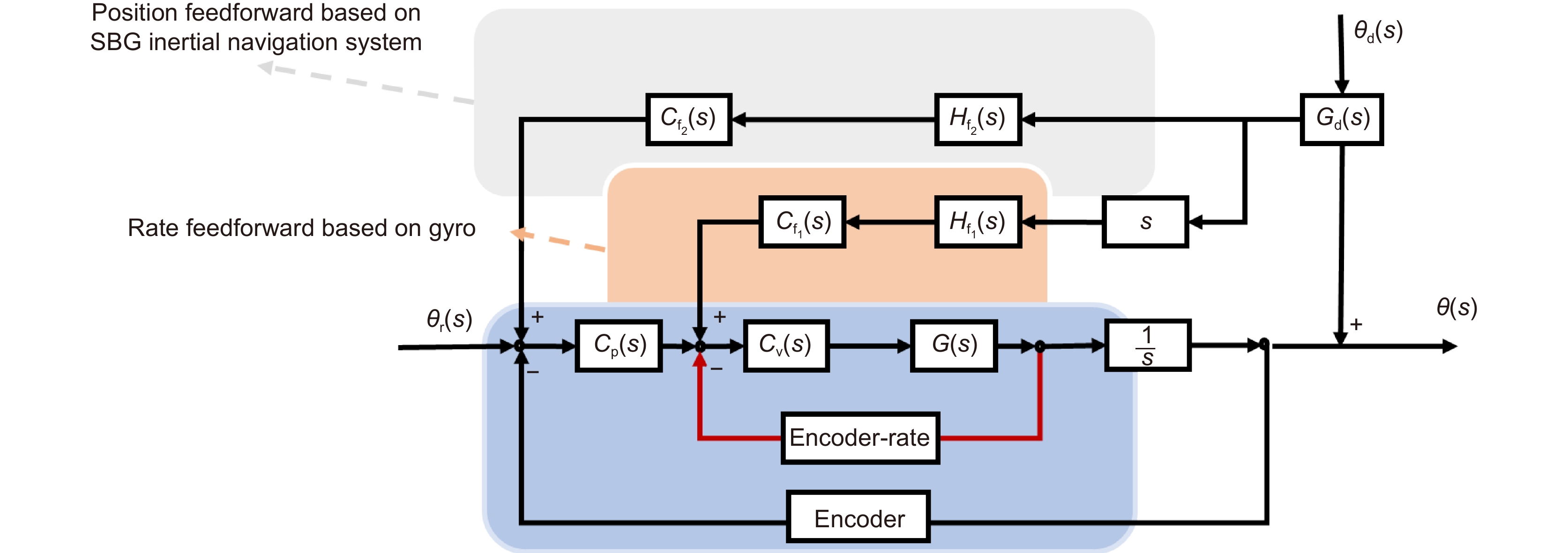
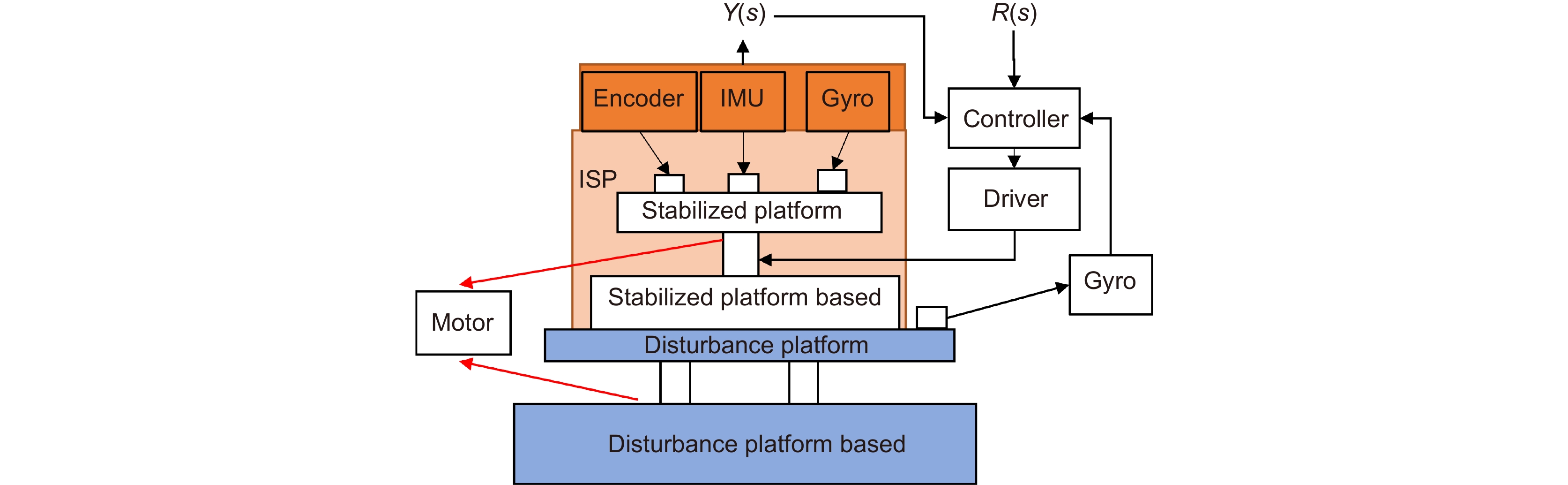
Overview: Inertial sensors are often used to maintain inertial stabilization and compensate disturbance in moving platform gimbal systems, which are widely used in engineering applications such as vehicles, satellites, ground-based equipment, and other moving platforms. Maintaining the line of sight (LOS) toward a target is challenging when the gimbal systems work in an environment with severe disturbance or when the target is highly dynamic. According to the installation methods of the sensors, the control methods can be divided into two types: direct stabilization and strapdown inertial stabilization. In direct stabilization method, the inertial sensors are directly mounted on the LOS axes to compensate the disturbance, which is simple to achieve but the disturbance suppression capability is limited by the closed-loop bandwidth of the system. However, the closed-loop bandwidth of the system is limited by the resonant frequency of the platform, which is difficult to improve, resulting in limited system disturbance surpression capability. The lightweight SBG inertial navigation system, produced by SBG Systems company, has the characteristics of being small and lightweight, which can be used for the direct and strapdown stabilization of the electro-optical tracking system. In this paper, five inertial stabilization control methods based on lightweight SBG navigation system in gimbals are studied, and theoretical analysis and experimental validation are performed. The classical position strapdown stabilization technique has limited disturbance suppression capability due to the limitation of position bandwidth, which makes it difficult to meet the requirement of high-precision stabilization. A SBG-based position-rate dual strapdown feedforward stabilization method is proposed, and the decoupling of the strapdown feedforward is achieved by introducing a high-pass filter, which can further improve the perturbation rejection bandwidth as well as the stabilization capability of the system. Theoretical analysis and experimental results show that the SBG-based gyro direct stabilization control method is better at disturbance suppression when the application conditions are not considered, although it is limited by the bandwidth. Under the limitations of platform volume and weight, the proposed SBG-based dual disturbance strapdown stabilization can further improve the system's disturbance rejection capability and obtain better stabilization accuracy.
-
-
图 5 基于SBG位置捷联稳定框图
Figure 5. Strapdown stabilization block diagram based on SBG position
图 6 基于SBG双扰动捷联控制框图
Figure 6. Dual disturbancetrapdown control block diagram based on SBG
图 7 惯性稳定万向架实验平台示意图
Figure 7. Inertial stabilization gimbal experimental platform diagram
图 8 不同频率扰动下三种直接稳定方法的系统误差
Figure 8. Stabilization errors under different disturbance frequencies
图 10 不同频率扰动下系统稳定误差及其傅里叶变换
Figure 10. Stabilization errors and Fourier transform under under different frequency disturbance
表 1 三种直接稳定方法的均方根误差对比
Table 1. RMS errors of three direct stabilization methods
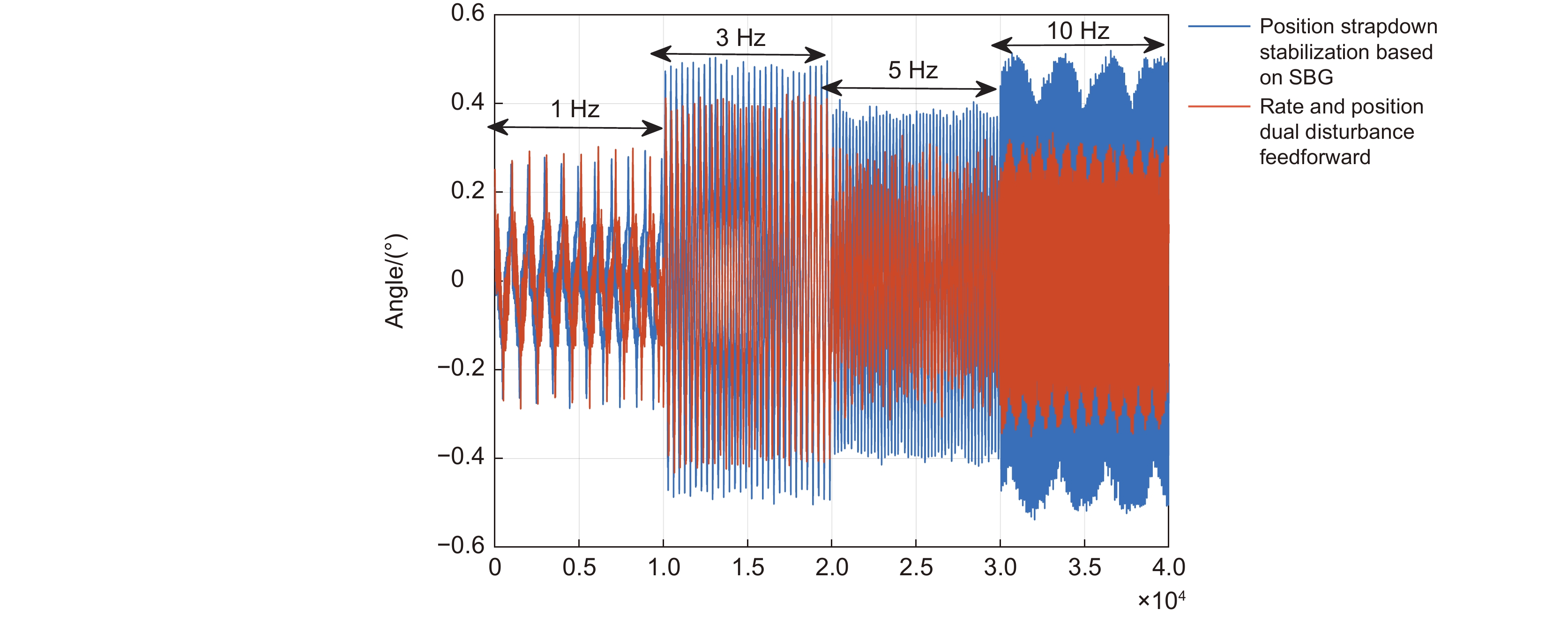
扰动频率/Hz 1 3 5 10 SBG直接反馈 0.0932 0.2490 0.3708 0.2616 基于SBG的速率反馈 0.0378 0.0816 0.0760 0.0461 基于SBG的陀螺前馈 0.0555 0.1087 0.1048 0.1294 
下载: 导出CSV
表 2 两种捷联稳定方法的均方根误差对比
Table 2. RMS errors of three direct stabilization methods
扰动频率/Hz 1 3 5 10 SBG捷联稳定 0.1074 0.2542 0.2335 0.3094 基于SBG双扰动捷联稳定 0.1024 0.2162 0.1758 0.1661
下载: 导出CSV
表 3 五种控制方法总结
Table 3. Summary of 5 control methods
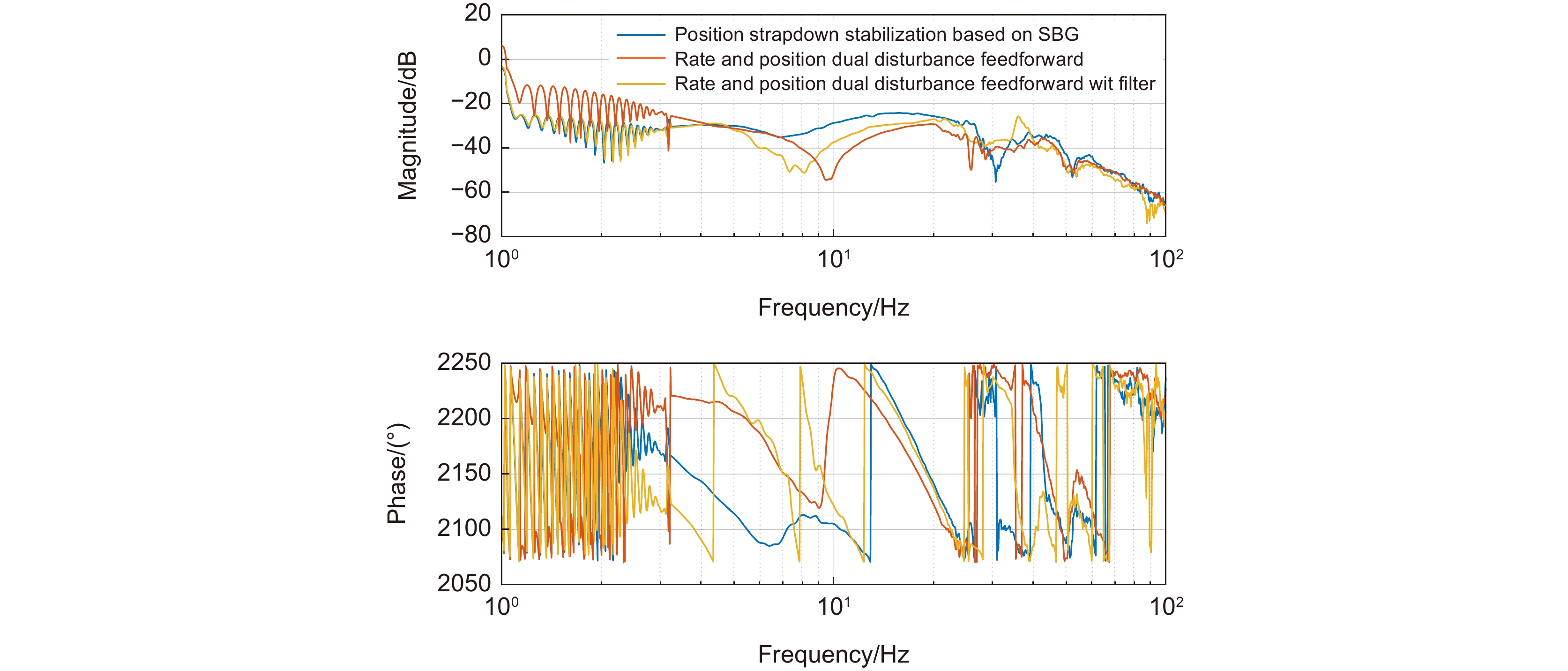
方法 扰动抑制能力 3 Hz 5 Hz 优缺点 适用场景 1 SBG直接稳定 −30 dB −26 dB 1)扰动抑制能力低，稳定精度差；
2)无需解算，可实现直接稳定对惯性姿态传感器体积、重量有要求 2 基于SBG反馈的速率反馈稳定 −42 dB −36 dB 1)扰动抑制能力较高，并且低频性能高；
2)陀螺安装在万向架；
3)可测量所有传递到万向架的扰动1)同方法1；
2)对陀螺体积、重量有要求3 基于SBG反馈的速率前馈稳定 −42 dB −36 dB 1)扰动抑制能力较高；
2)利用平台速率信息，
但无法测量万向架内部扰动1)同方法1；
2)平台需要提供速率信息4 基于SBG的位置捷联稳定 −32 dB −30 dB 1)扰动抑制带宽低，稳定精度较差；
2)无法测量万向架的扰动经典捷联稳定 5 基于SBG双扰动捷联稳定 −34 dB −32 dB 1)扰动抑制带宽高，稳定精度好；
2)需要扰动解耦；
3)无法测量万向架的扰动经典捷联稳定以及速率前馈
下载: 导出CSV
-
[1] Yu Z Y, Yang T, Ruan Y, et al. A rate-difference disturbance observer control for a timing-belt servo system[J]. IEEE Trans Ind Electron, 2022, 69(1): 11458−11467. doi: 10.1109/tie.2021.3123642
[2] Hurák Z, Rezac M. Image-based pointing and tracking for inertially stabilized airborne camera platform[J]. IEEE Trans Control Syst Technol, 2012, 20(5): 1146−1159. doi: 10.1109/TCST.2011.2164541
[3] Ruan Y, Xu T R, Liu Y, et al. Error-based observation control of an image-based control loop for disturbance suppression in segmented lightweight large-scaled diffractive telescope (SLLDT)[J]. Opt Lasers Eng, 2022, 156: 107105. doi: 10.1016/j.optlaseng.2022.107105
[4] 唐涛, 马佳光, 陈洪斌, 等. 光电跟踪系统中精密控制技术研究进展[J]. 光电工程, 2020, 47(10): 200315. doi: 10.12086/oee.2020.200315
Tang T, Ma J G, Chen H B, et al. A review on precision control methodologies for optical-electric tracking control system[J]. Opto-Electron Eng, 2020, 47(10): 200315. doi: 10.12086/oee.2020.200315
[5] 李志俊, 毛耀, 亓波, 等. 量子光通信中位置修正单检测控制方法[J]. 光电工程, 2022, 49(3): 210311. doi: 10.12086/oee.2022.210311
Li Z J, Mao Y, Qi B, et al. Research on control technology of single detection based on position correction in quantum optical communication[J]. Opto-Electron Eng, 2022, 49(3): 210311. doi: 10.12086/oee.2022.210311
[6] Hilkert J M. Inertially stabilized platform technology concepts and principles[J]. IEEE Control Syst Mag, 2008, 28(1): 26−46. doi: 10.1109/MCS.2007.910256
[7] 张良总, 杨涛, 吴云, 等. 基于图像测量的Stewart平台双阶控制技术[J]. 光电工程, 2022, 49(8): 220019. doi: 10.12086/oee.2022.220019
Zhang L Z, Yang T, Wu Y, et al. Image measurement-based two-stage control of Stewart platform[J]. Opto-Electron Eng, 2022, 49(8): 220019. doi: 10.12086/oee.2022.220019
[8] 田竞. 惯性稳定平台中的多传感器控制技术研究[D]. 成都: 电子科技大学, 2016.
Tian J. Research on multiple sensors control technology of inertial stabled-platforms[D]. Chengdu: University of Electronic Science and Technology of China, 2016.
[9] Glück M, Pott J U, Sawodny O. Investigations of an accelerometer-based disturbance feedforward control for vibration suppression in adaptive optics of large telescopes[J]. Publ Astron Soc Pac, 2017, 129(976): 065001. doi: 10.1088/1538-3873/aa632b
[10] El-Sheimy N, Youssef A. Inertial sensors technologies for navigation applications: state of the art and future trends[J]. Satell Navig, 2020, 1(1): 2. doi: 10.1186/s43020-019-0001-5
[11] 夏文强, 何秋农, 段倩文, 等. 基于传感器优化与鲁棒预测的等效加速度前馈[J]. 光电工程, 2021, 48(11): 210153. doi: 10.12086/oee.2021.210153
Xia W Q, He Q N, Duan Q W, et al. Equivalent acceleration feedforward based on sensor optimization and robust prediction[J]. Opto-Electron Eng, 2021, 48(11): 210153. doi: 10.12086/oee.2021.210153
[12] Řezáč M, Hurák Z. Vibration rejection for inertially stabilized double gimbal platform using acceleration feedforward[C]//2011 IEEE International Conference on Control Applications, 2011: 363–368. https://doi.org/10.1109/CCA.2011.6044442.
[13] Li M, Li J, Zhang R Q. Unbalance disturbance restraining for inertial stabilized platform[C]//2016 Chinese Control and Decision Conference, 2016: 2723–2728. https://doi.org/10.1109/CCDC.2016.7531444.
[14] Xia Y Z, Bao Q L, Liu Z D. A new disturbance feedforward control method for electro-optical tracking system line-of-sight stabilization on moving platform[J]. Sensors, 2018, 18(12): 4350. doi: 10.3390/s18124350
[15] Kennedy P J, Kennedy R L. Direct versus indirect line of sight (LOS) stabilization[J]. IEEE Trans Control Syst Technol, 2003, 11(1): 3−15. doi: 10.1109/TCST.2002.806443
[16] Sisu S, Şuvak Ö. Comparison of direct and indirect stabilization methods for gyro stabilized gimbal systems[C]//2020 12th International Conference on Electrical and Electronics Engineering, 2020: 251–255.
[17] Chen Y, Xue Y Y, Zhang X J, et al. Target tracking system based on inertial stabilized platform[J]. Appl Mech Mater, 2012, 271–272: 1669−1674. doi: 10.4028/www.scientific.net/AMM.271-272.1669
[18] Zubov A V. Stabilization of program motion and kinematic trajectories in dynamic systems in case of systems of direct and indirect control[J]. Autom Remote Control, 2007, 68(3): 386−398. doi: 10.1134/S0005117907030022
-
点击扫一扫
图(13)
表(3)
计量
- 文章访问数:
- PDF下载数:
- 施引文献: 0
