E-mail Alert
RSS
-
摘要:
本文立足于太赫兹波成像领域近年来备受关注的研究热点—太赫兹波计算鬼成像，首先回顾了鬼成像从量子到经典再到计算的历史过程，然后阐述了计算鬼成像的数学原理，随后综述了计算鬼成像在太赫兹波段的发展历程，及其在超衍射分辨成像、石墨烯光电导成像、太赫兹光谱成像等方面的应用，并在最后展望了太赫兹波计算鬼成像的发展前景：计算鬼成像作为一种成像手段，可以绕开在太赫兹频段缺乏经济高效的焦面阵列式探测器的难题，但目前的成像帧率还难以满足快速成像的应用需求，相信在未来随着器件性能的提升和成像算法的优化，其成像帧率可以得到大幅提升。
Abstract:Based on a research hotspot in field of terahertz (THz) wave imaging—THz wave ghost imaging, we first reviewed the development history of ghost imaging: from quantum to classical and then to computational. Second, the mathematical principles of the computational ghost imaging were described in details. Then, we reviewed the development history of computational ghost imaging within THz regime, and its applications including sub-diffraction imaging, photoconductivity mapping of graphene, and hyperspectral THz imaging. At last, we looked towards the prospects of THz wave ghost imaging: as an imaging scheme, ghost imaging avoids the problem that economic and efficient focal-plane-array detectors within THz regime are lacking, whose present frame rates, however, are too slow to meet the requirement for fast imaging. With the improvement of devices' performance and the optimization of imaging algorithms, we believe that the frame rate of THz wave computational ghost imaging can be significantly enhanced in the future.
-
Key words:
- ghost imaging /
- single-pixel imaging /
- correlated imaging /
- terahertz waves
-
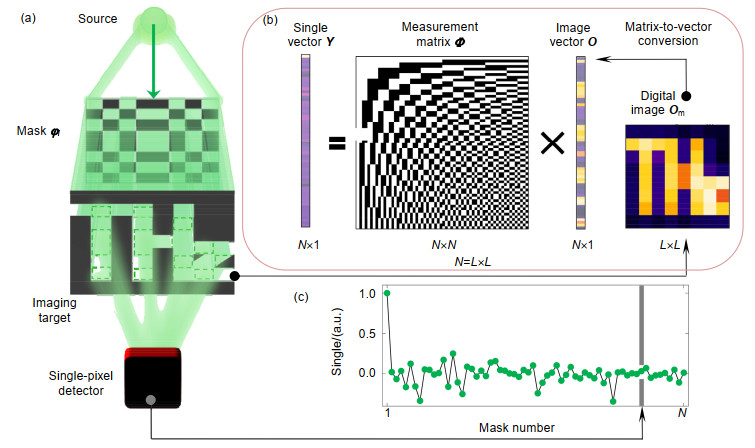
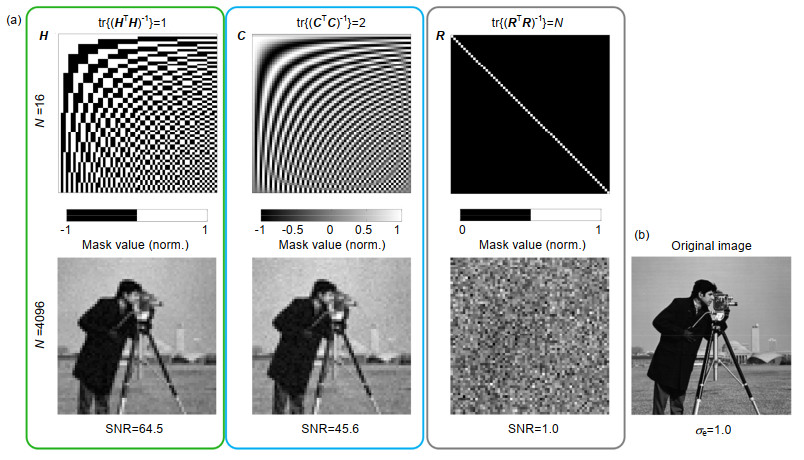
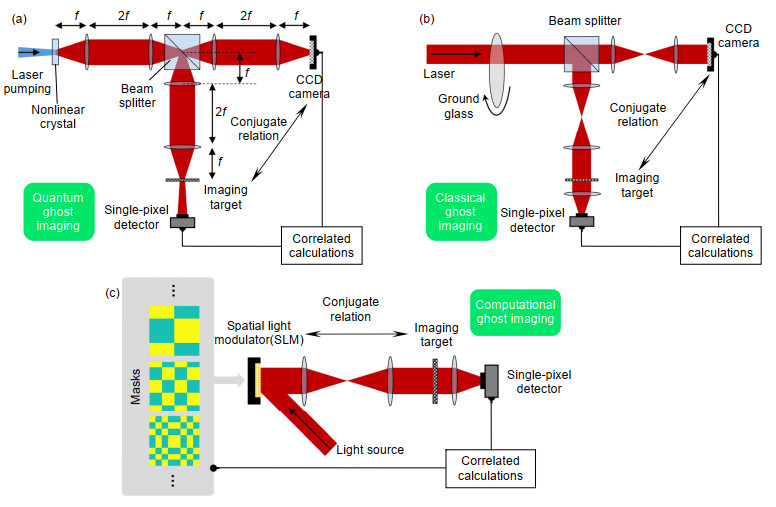
Overview: Distinguishing from the widely used focal-plane-array imaging (e.g., the charge coupled device, CCD), another novel imaging scheme, computational ghost imaging (which is also called single-pixel imaging or correlated imaging) acquires object's image by computing the correlations between the varied illuminating field and the imaging target with a no-spatial-resolution detector (single-pixel detector). Comparing with the well-developed silicon-based focal-plane-array cameras, computational ghost imaging is simpler, smaller, and, most significantly, can operate efficiently across a much broader spectral range. Moreover, this imaging methodology can be combined with some novel acquisition technologies, such as compressed sensing and adaptive imaging, making it suitable for many specific imaging applications. On the other hand, terahertz (THz) waves, covering the frequencies ranging from 0.1 THz to 10 THz, have many unique properties, such as the spectral fingerprint, high transmittance in most polar materials, high absorption by water, non-ionizing photon energy (1 THz, 4 meV). There are a lot of applications within THz waves, covering the fields of medical and biology sciences, non-destructive detection, security check, high-speed wireless communication and so on. Imaging with THz waves is also significant in many situations. However, since the focal-plane-array detector available within THz waves is expensive or complicated to fabricate, the main method for THz wave imaging was usually based on raster scanning. The computational ghost imaging within THz waves was firstly demonstrated in 2008, which paves a new route for THz wave imaging and inspires many applications including sub-diffraction-limit THz wave imaging and spatially resolved photoconductivity of graphene. In this article, we firstly review the historical developing process of ghost imaging, namely from the quantum ghost imaging to classical ghost imaging and then to computational ghost imaging. Secondly, the computational ghost imaging is described mathematically in details, including the linear mapping during the imaging process, algorithm for recovering the ghost image and a discussion about the performance of various measurement matrices in noisy imaging environment. And then, we introduce several computational ghost imaging applications within THz waves, including the first demonstration of THz wave computational ghost imaging, the invention of the dynamic spatial THz wave modulator, the sub-diffraction-limit THz wave computational ghost imaging and the photoconductivity mapping of graphene in THz region. At last, we outlook the prospects of the THz wave computational ghost imaging. We hope this review article can help the readers better understand the principles, applications, and prospects of the THz wave computational ghost imaging.
-
-
图 1 (a) 量子鬼成像示意图；(b)经典鬼成像示意图；(c)计算鬼成像示意图
Figure 1. Schematics of (a) quantum ghost imaging, (b) classical ghost imaging, and (c) computational ghost imaging
图 2 (a) 计算鬼成像的单次关联测量示意图；(b)计算鬼成像的数学描述；(c)测量值向量Y(示例)
Figure 2. (a) Schematic of a single computational ghost measurement; (b) Computational ghost imaging described in mathematic; (c) An example of measurement vector Y
图 3 常用观测矩阵及其在噪声环境下的鬼成像仿真结果
Figure 3. The common observation matrix and simulation results of ghost imaging in noisy environment
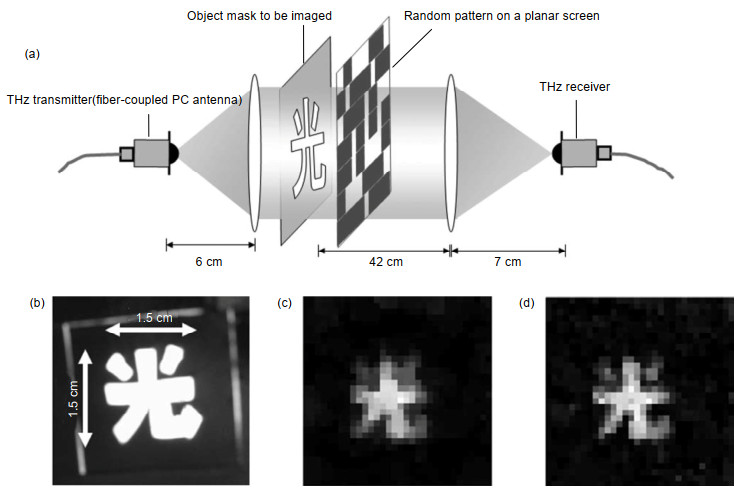
图 4 莱斯大学实现太赫兹波段计算鬼成像。(a)实验示意图；(b)~(d)鬼成像结果[33]。(b)为成像目标的光学图像，(c)和(d)分别是采样率为30%和60%情况下的，基于最小变分法重构的压缩图像(0.1 THz)，像素大小为1 mm，像素阵列为32×32
Figure 4. First demonstration of THz computational ghost imaging[33]. (a) Schematic of the THz computational ghost imaging; (b) The photography of the imaging target; The compressive THz ghost images with sample ratio of 30% (c) and 60% (d)
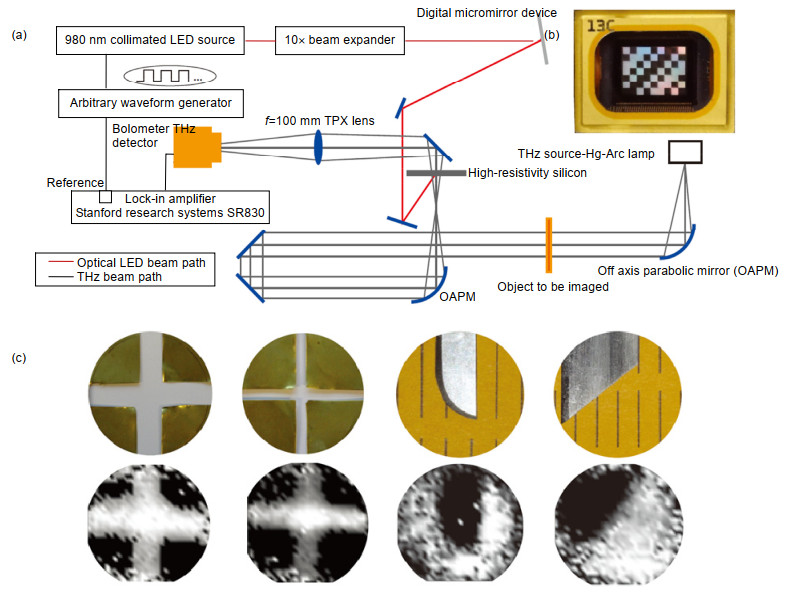
图 5 Padilla团队利用动态可重构的STWM实现太赫兹计算鬼成像[34]。(a)实验示意图；(b) DMD光学照片；(c)成像目标光学照片(上)及相应的太赫兹计算鬼成像结果(下)
Figure 5. THz computational ghost imaging using dynamic spatial THz wave modulator[34]. (a) Schematic of the experimental optical layouts; (b) Photography of the DMD; (c) Four imaging targets (upper) and the corresponding THz computational ghost images (lower)
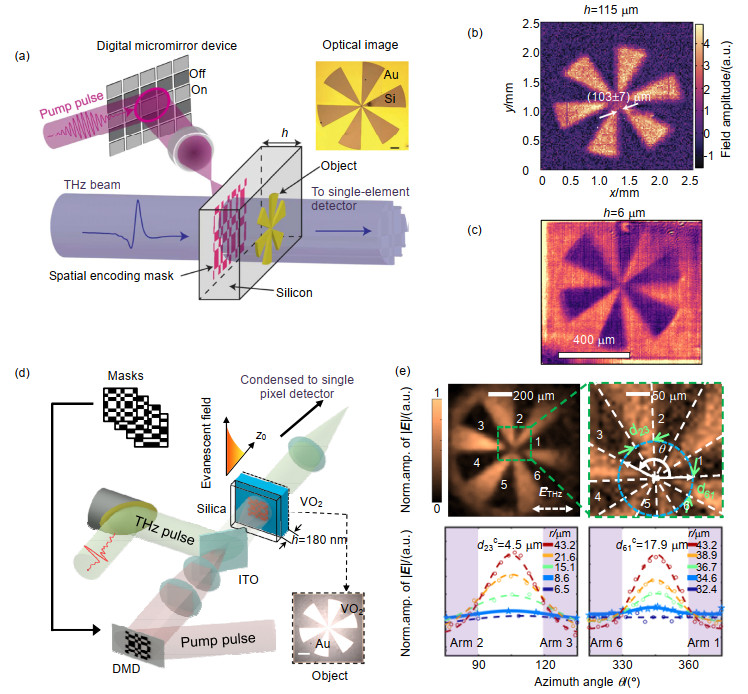
图 6 超衍射极限分辨的太赫兹计算鬼成像。(a)基于空间光泵浦Si的实验示意图；(b), (c)在Si片厚度分别为115 μm[37]和6 μm[38]时的太赫兹超分辨计算鬼成像结果；(d), (e)分别是基于空间光泵浦VO2的实验示意图及其太赫兹超分辨计算鬼成像结果[41]
Figure 6. Sub-diffraction-limited THz computational ghost imaging. (a) The system based on spatial light pumping Si; (b), (c) The resultant THz ghost images with 115 μm thick Si[37] and 6 μm thick Si[38], respectively; (d) The system based on spatial light pumping VO2; (e) The resultant THz ghost images[41]
图 7 空间采样以实现超衍射分辨的太赫兹波计算鬼成像[44]。(a)采样方法示意图；(b)成像目标示意(左)及太赫兹波成像实验结果(右)
Figure 7. Sub-diffraction-limited THz wave computational ghost imaging realized via spatial sampling[44]. (a) Schematic of the spatial sampling methodology; (b) The imaging target (left panel) and the corresponding THz wave imaging results (right panel)
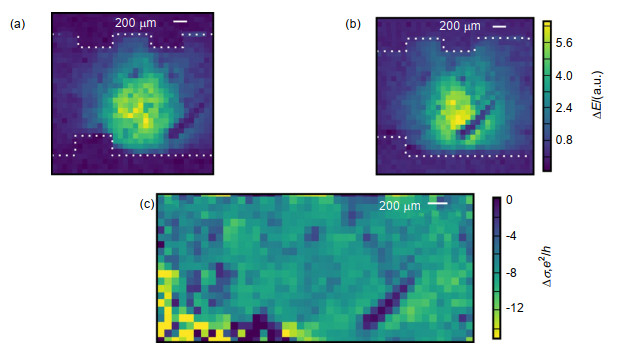
图 8 石墨烯光电导分布的太赫兹计算鬼成像[45]。(a)光泵浦石墨烯在某个视场下导致的太赫兹场透射场强变化空间分布∆E；(b)石墨烯横向移动了450 μm后的∆E；(c)计算出的石墨烯在太赫兹频段的光电导变化的空间分布∆σ。(a~c)中可以明显观察到石墨烯有一处缺陷
Figure 8. THz response to graphene's photoconductivity distribution acquired by computational ghost imaging[45]. (a) The spatial dependence of ∆E under some certain field of view. The ∆E is defined as ∆E=EPumpOn-EPumpOff, where EPumpOn and EPumpOff are the transmitted THz field peak amplitude before and after optically pumping, respectively; (b) ∆E measured with a horizontal shift of 450 μm with respect to panel (a); (c) The calculated spatial dependence of the graphene's THz photoconductivity ∆σ. One can observe a defect in the graphene in (a~c)
图 9 由TNGI技术获得的目标太赫兹光谱图像[46]。(a)成像目标的示意图，其中黄色部分表示金，蓝色部分表示teflon，红色部分表示kapton；(b)~(d)目标在不同频点的太赫兹图像；(e)厚度为10 μm的teflon(蓝色)和kapton(红色)的太赫兹透射幅度谱，其中黑色点线表示入射太赫兹幅度频谱；(f)不同频点处光谱图像的SSIM (structural similarity index method)。(b)~(d)所对应的频点在(e)和(f)中用对应的红色垂直虚线标出
Figure 9. Hyperspectral imaging via time-resolved nonlinear ghost imaging (TNGI)[46]. (a) Schematic of the imaging target; (b)~(d) Ghost images for different chemical selectivity; (e) Transmission spectrum for teflon (blue) and kapton (red) both with thickness of 10 μm. The black dotted line indicates the THz source spectrum; (f) The frequency-dependent SSIM (Structural similarity index method) analysis of the hyperspectral image. The red vertical dashed lines labeled by b, c and d both in (e) and (f) correspond to the hyperspectral images (b), (c) and (d), respectively
-
[1] Erkmen B I, Shapiro J H. Ghost imaging: from quantum to classical to computational[J]. Advances in Optics and Photonics, 2010, 2(4): 405-450. doi: 10.1364/AOP.2.000405
[2] Brown R H, Twiss R Q. Correlation between photons in two coherent beams of light[J]. Nature, 1956, 177(4497): 27-29. doi: 10.1038/177027a0
[3] Brown R H, Twiss R Q. A test of a new type of stellar interferometer on sirius[J]. Nature, 1956, 178(4541): 1046-1048. doi: 10.1038/1781046a0
[4] Pittman T B, Shih Y H, Strekalov D V, et al. Optical imaging by means of two-photon quantum entanglement[J]. Physical Review A, 1995, 52(5): R3429-R3432. doi: 10.1103/PhysRevA.52.R3429
[5] Abouraddy A F, Saleh B E A, Sergienko A V, et al. Role of entanglement in two-photon imaging[J]. Physical Review Letters, 2001, 87(12): 123602. doi: 10.1103/PhysRevLett.87.123602
[6] Bennink R S, Bentley S J, Boyd R W. "Two-Photon" coincidence imaging with a classical source[J]. Physical Review Letters, 2002, 89(11): 113601. doi: 10.1103/PhysRevLett.89.113601
[7] Shapiro J H. Computational ghost imaging[J]. Physical Review A, 2008, 78(6): 061802. doi: 10.1103/PhysRevA.78.061802
[8] Duarte M F, Davenport M A, Takhar D, et al. Single-pixel imaging via compressive sampling[J]. IEEE Signal Processing Magazine, 2008, 25(2): 83-91. doi: 10.1109/MSP.2007.914730
[9] Candes E J, Romberg J, Tao T. Robust uncertainty principles: exact signal reconstruction from highly incomplete frequency information[J]. IEEE Transactions on Information Theory, 2006, 52(2): 489-509. doi: 10.1109/TIT.2005.862083
[10] Candes E J, Tao T. Near-optimal signal recovery from random projections: universal encoding strategies?[J]. IEEE Transactions on Information Theory, 2006, 52(12): 5406-5425. doi: 10.1109/TIT.2006.885507
[11] Donoho D L. Compressed sensing[J]. IEEE Transactions on Information Theory, 2006, 52(4): 1289-1306. doi: 10.1109/TIT.2006.871582
[12] Katz O, Bromberg Y, Silberberg Y. Compressive ghost imaging[J]. Applied Physics Letters, 2009, 95(13): 131110. doi: 10.1063/1.3238296
[13] Bennink R S, Bentley S J, Boyd R W, et al. Quantum and classical coincidence imaging[J]. Physical Review Letters, 2004, 92(3): 033601. doi: 10.1103/PhysRevLett.92.033601
[14] Zhang P L, Gong W L, Shen X, et al. Improving resolution by the second-order correlation of light fields[J]. Optics Letters, 2009, 34(8): 1222-1224. doi: 10.1364/OL.34.001222
[15] Harwit M, Sloane N J A. Hadamard Transform Optics[M]. New York: Academic Press, 1979.
[16] Tonouchi M. Cutting-edge terahertz technology[J]. Nature Photonics, 2007, 1(2): 97-105. doi: 10.1038/nphoton.2007.3
[17] Meng K, Chen T N, Chen T, et al. Terahertz pulsed spectroscopy of paraffin-embedded brain glioma[J]. Journal of Biomedical Optics, 2014, 19(7): 077001. doi: 10.1117/1.JBO.19.7.077001
[18] Zou Y, Li J, Cui Y Y, et al. Terahertz spectroscopic diagnosis of myelin deficit brain in mice and rhesus monkey with chemometric techniques[J]. Scientific Reports, 2017, 7(1): 5176. doi: 10.1038/s41598-017-05554-z
[19] Zou Y, Liu Q, Yang X, et al. Label-free monitoring of cell death induced by oxidative stress in living human cells using terahertz ATR spectroscopy[J]. Biomedical Optics Express, 2018, 9(1): 14-24. doi: 10.1364/BOE.9.000014
[20] Hu B B, Nuss M C. Imaging with terahertz waves[J]. Optics Letters, 1995, 20(16): 1716-1718. doi: 10.1364/OL.20.001716
[21] Tang P R, Li J, Du L H, et al. Ultrasensitive specific terahertz sensor based on tunable plasmon induced transparency of a graphene micro-ribbon array structure[J]. Optics Express, 2018, 26(23): 30655-30666. doi: 10.1364/OE.26.030655
[22] Van Exter M, Fattinger C, Grischkowsky D. Terahertz time-domain spectroscopy of water vapor[J]. Optics Letters, 1989, 14(20): 1128-1130. doi: 10.1364/OL.14.001128
[23] Nakajima S, Hoshina H, Yamashita M, et al. Terahertz imaging diagnostics of cancer tissues with a chemometrics technique[J]. Applied Physics Letters, 2007, 90(4): 041102. doi: 10.1063/1.2433035
[24] Koenig S, Lopez-Diaz D, Antes J, et al. Wireless sub-THz communication system with high data rate[J]. Nature Photonics, 2013, 7(12): 977-981. doi: 10.1038/nphoton.2013.275
[25] Nagatsuma T, Ducournau G, Renaud C C. Advances in terahertz communications accelerated by photonics[J]. Nature Photonics, 2016, 10(6): 371-379. doi: 10.1038/nphoton.2016.65
[26] Tang H, Zhu L G, Zhao L, et al. Carrier dynamics in Si nanowires fabricated by metal-assisted chemical etching[J]. ACS Nano, 2012, 6(9): 7814-7819. doi: 10.1021/nn301891s
[27] Xiao Y, Zhai Z H, Shi Q W, et al. Ultrafast terahertz modulation characteristic of tungsten doped vanadium dioxide nanogranular film revealed by time-resolved terahertz spectroscopy[J]. Applied Physics Letters, 2015, 107(3): 031906. doi: 10.1063/1.4927383
[28] Zhai Z H, Zhu H F, Shi Q, et al. Enhanced photoresponses of an optically driven VO2-based terahertz wave modulator near percolation threshold[J]. Applied Physics Letters, 2018, 113(23): 231104. doi: 10.1063/1.5050681
[29] Zhai Z H, Chen S C, Du L H, et al. Giant impact of self-photothermal on light-induced ultrafast insulator-to-metal transition in VO2 nanofilms at terahertz frequency[J]. Optics Express, 2018, 26(21): 28051-28066. doi: 10.1364/OE.26.028051
[30] Karpowicz N, Zhong H, Zhang C L, et al. Compact continuous-wave subterahertz system for inspection applications[J]. Applied Physics Letters, 2005, 86(5): 054105. doi: 10.1063/1.1856701
[31] Charron D M, Ajito K, Kim J Y, et al. Chemical mapping of pharmaceutical cocrystals using terahertz spectroscopic imaging[J]. Analytical Chemistry, 2013, 85(4): 1980-1984. doi: 10.1021/ac302852n
[32] Yang X, Zhao X, Yang K, et al. Biomedical applications of terahertz spectroscopy and imaging[J]. Trends in Biotechnology, 2016, 34(10): 810-824. doi: 10.1016/j.tibtech.2016.04.008
[33] Chan W L, Charan K, Takhar D, et al. A single-pixel terahertz imaging system based on compressed sensing[J]. Applied Physics Letters, 2008, 93(12): 121105. doi: 10.1063/1.2989126
[34] Shrekenhamer D, Watts C M, Padilla W J. Terahertz single pixel imaging with an optically controlled dynamic spatial light modulator[J]. Optics Express, 2013, 21(10): 12507-12518. doi: 10.1364/OE.21.012507
[35] Watts C M, Shrekenhamer D, Montoya J, et al. Terahertz compressive imaging with metamaterial spatial light modulators[J]. Nature Photonics, 2014, 8(8): 605-609. doi: 10.1038/nphoton.2014.139
[36] Landy N I, Sajuyigbe S, Mock J J, et al. Perfect metamaterial absorber[J]. Physical Review Letters, 2008, 100(20): 207402. doi: 10.1103/PhysRevLett.100.207402
[37] Stantchev R I, Sun B Q, Hornett S M, et al. Noninvasive, near-field terahertz imaging of hidden objects using a single-pixel detector[J]. Science Advances, 2016, 2(6): e1600190. doi: 10.1126/sciadv.1600190
[38] Stantchev R I, Phillips D B, Hobson P, et al. Compressed sensing with near-field THz radiation[J]. Optica, 2017, 4(8): 989-992. doi: 10.1364/OPTICA.4.000989
[39] Aβmann M, Bayer M. Compressive adaptive computational ghost imaging[J]. Scientific Reports, 2013, 3: 1545. doi: 10.1038/srep01545
[40] Green M A. Self-consistent optical parameters of intrinsic silicon at 300 K including temperature coefficients[J]. Solar Energy Materials and Solar Cells, 2008, 92(11): 1305-1310. doi: 10.1016/j.solmat.2008.06.009
[41] Chen S C, Du L H, Meng K, et al. Terahertz wave near-field compressive imaging with a spatial resolution of over λ/100[J]. Optics Letters, 2019, 44(1): 21-24. doi: 10.1364/OL.44.000021
[42] Liu K, Lee S, Yang S, et al. Recent progresses on physics and applications of vanadium dioxide[J]. Materials Today, 2018, 21(8): 875-896. doi: 10.1016/j.mattod.2018.03.029
[43] Cavalleri A, Tóth C, Siders C W, et al. Femtosecond structural dynamics in VO2 during an ultrafast solid-solid phase transition[J]. Physical Review Letters, 2001, 87(23): 237401. doi: 10.1103/PhysRevLett.87.237401
[44] Zhao J P, E Y W, Williams K, et al. Spatial sampling of terahertz fields with sub-wavelength accuracy via probe-beam encoding[J]. Light: Science & Applications, 2019, 8(1): 55. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=gkxyyy-e201904010
[45] Hornett S M, Stantchev R I, Vardaki M Z, et al. Subwavelength terahertz imaging of graphene photoconductivity[J]. Nano Letters, 2016, 16(11): 7019-7024. doi: 10.1021/acs.nanolett.6b03168
[46] Olivieri L, Totero Gongora J S, Pasquazi A, et al. Time-resolved nonlinear ghost imaging[J]. ACS Photonics, 2018, 5(8): 3379-3388. doi: 10.1021/acsphotonics.8b00653
[47] Olivieri L, Gongora J S T, Peters L, et al. Hyperspectral terahertz microscopy via nonlinear ghost imaging[J]. Optica, 2020, 7(2): 186-191. doi: 10.1364/OPTICA.381035
-
下载:
点击扫一扫
图(9)
计量
- 文章访问数:
- PDF下载数:
- 施引文献: 0
