Characteristic analysis of orbital angular momentum of vortex beam propagating in atmospheric turbulent
-
摘要:
从拉盖尔-高斯涡旋光束表达式出发,基于瑞利衍射理论,通过研究涡旋光束在大气湍流中传输时的旋转相干函数的变化规律,总结了涡旋光束在大气湍流中传输时各轨道角动量之间的串扰情况,使用了拓扑荷数探测概率描述串扰规律,并推导了拓扑荷数探测概率的解析表达式。研究了涡旋光束通过湍流后的拓扑荷数的分布情况,并将结果与涡旋光束通过大气随机相位屏的数值仿真结果进行了对比,给出了理论与仿真的拓扑荷数的探测概率随湍流强度以及初始涡旋光束拓扑荷数大小的关系图对比,验证了推导的拓扑荷数探测概率解析表达式的正确性。通过该表达式可进一步研究大气湍流与涡旋光束相互作用从而影响涡旋光束轨道角动量散射的本质,为涡旋光束的空间光通信中选择合适的拓扑荷数间隔,以及在不同湍流强度下选择合适束腰大小以减少串扰带来的误码率提供了理论依据。
 Abstract:
Abstract:Starting from the expression of Laguerre-Gaussian vortex beam and based on Rayleigh diffraction theory, the variation of rotating coherence function of vortex beam propagating in atmospheric turbulence is studied. The crosstalk between the angular momentum of each orbital angular momentum when the vortex beam propagates in atmospheric turbulence is summarized. The topological charge detection probability is used to describe the crosstalk law, and the analytical expression of the topological charge detection probability is derived. The distribution of topological charge number of vortex beam passing through turbulence is studied, and the results are compared with the numerical simulation results of vortex beam passing through atmospheric random phase screen. The relationship between the detection probability of the theoretical and simulated topological charge numbers with the turbulence intensity and the topological charge number of the initial vortex beam is compared, and the correctness of the analytical expression of the topological charge number detection probability is verified. Through this expression, the interaction between atmospheric turbulence and vortex beam can be further studied, which can affect the essence of angular momentum scattering of vortex beam, and the suitable topological charge number interval can be selected for the space optical communication of vortex beam. It also provides a theoretical basis for selecting the appropriate beam waist size under different turbulence intensities to reduce the bit error rate (BER) caused by crosstalk.
-
Key words:
- atmospheric turbulence /
- vortex beam /
- topological charge /
- orbital angular momentum
-

Overview: In recent years, with the deepening of the study of the propagation characteristics of all kinds of beams, a vortex beam with a new phase structure has been gradually discovered and has become a research focus because of its novel characteristics. The central light intensity of the vortex beam is zero, the phase structure of the wavefront is spiral, and there is a phase singularity in the center of the beam. This spiral phase structure makes the vortex beam have orbital angular momentum, which provides a new channel reuse dimension for space optical communication and improves the channel capacity. However, when the vortex beam passes through the atmospheric turbulence, the intensity and phase distribution of the beam will be affected by the turbulence, which will further cause crosstalk between the angular momentum of each orbit. Finally, the increase of bit error rate (BER) and the decrease of communication capacity are caused by the increase of bit error rate and the decrease of communication capacity. Therefore, the study of the factors affecting the topological charge number scattering of vortex beams and the crosstalk of orbital angular momentum is of great significance for the further study of the interaction between orbital angular momentum and atmospheric turbulence, and is beneficial to improve the capacity of space optical communication system. Starting from the expression of Laguerre-Gaussian vortex beam and based on Rayleigh diffraction theory, the variation of rotating coherence function of vortex beam propagating in atmospheric turbulence is studied. The crosstalk between the angular momentum of each orbital angular momentum when the vortex beam propagates in atmospheric turbulence is summarized. The topological charge detection probability is used to describe the crosstalk law, and the analytical expression of the topological charge detection probability is derived. The distribution of topological charge number of vortex beam passing through turbulence is studied, and the results are compared with the numerical simulation results of vortex beam passing through atmospheric random phase screen. The relationship between the detection probability of the theoretical and simulated topological charge numbers with the turbulence intensity and the topological charge number of the initial vortex beam is compared, and the correctness of the analytical expression of the topological charge number detection probability is verified. Through this expression, the interaction between atmospheric turbulence and vortex beam can be further studied, which can affect the essence of angular momentum scattering of vortex beam, and the suitable topological charge number interval can be selected for the space optical communication of vortex beam. It also provides a theoretical basis for selecting the appropriate beam waist size under different turbulence intensities to reduce the bit error rate caused by crosstalk.
-

-
图 2 涡旋光束拓扑荷数为3,大气折射率结构常数Cn2=1×10-14 m-2/3时,涡旋光束在源平面处的光强分布(a)和相位分布(b);通过大气湍流后接受面上的光强分布(c)和相位分布(d);(e)数值计算的各拓扑荷数的探测概率;(f)采用式(16)计算的各拓扑荷数的理论探测概率
Figure 2. The topological charge of the vortex beam is 3, the refractive index structure constant of atmospheric turbulence is Cn2=1×10-14 m-2/3. Intensity distribution (a) and the phase (b) of the vortex beam at the source plane; The intensity distribution (c) and the phase (d) on the receiving plane; (e) Probability of detection of each topological charge obtained by numerical calculation; (f) Probability of detection of each topological charge calculated by equation (16)
图 3 左列表示原始拓扑荷数为1时,不同湍流强度下涡旋光束传输40次后的各拓扑荷数的平均探测概率,右列表示相应拓扑荷数的平均探测概率和理论探测概率的对比。(a~b)大气折射率结构常数为Cn2=1×10-14 m-2/3;(c~d)大气折射率结构常数为Cn2=5×10-14 m-2/3;(e~f)大气折射率结构常数为Cn2=1×10-13 m-2/3
Figure 3. The topological charge of the vortex beam is 1, the average detection probability of each topological charge after 40 times of vortex beam transmission at different turbulence and the comparison between the average detection probability and the theoretical detection probability of the corresponding topological charge. (a~b) The refractive index structure constant of atmospheric turbulence is Cn2=1×10-14 m-2/3; (c~d) The refractive index structure constant of atmospheric turbulence is Cn2=5×10-14 m-2/3; (e~f) The refractive index structure constant of atmospheric turbulence is Cn2=1×10-13 m-2/3
图 4 左列表示原始拓扑荷数为3时,不同湍流强度下涡旋光束传输40次后的各拓扑荷数的平均探测概率,右列表示相应拓扑荷数的平均探测概率和理论探测概率的对比。(a~b)大气折射率结构常数为Cn2=1×10-14 m-2/3;(c~d)大气折射率结构常数为Cn2=5×10-14 m-2/3;(e~f)大气折射率结构常数为Cn2=1×10-13 m-2/3。
Figure 4. The topological charge of the vortex beam is 3, the average detection probability of each topological charge after 40 times of vortex beam transmission at different turbulence and the comparison between the average detection probability and the theoretical detection probability of the corresponding topological charge. (a~b) The refractive index structure constant of atmospheric turbulence is Cn2=1×10-14 m-2/3; (c~d) The refractive index structure constant of atmospheric turbulence is Cn2=5×10-14 m-2/3; (e~f) The refractive index structure constant of atmospheric turbulence is Cn2=1×10-13 m-2/3.
图 5 左列表示原始拓扑荷数为9时,不同湍流强度下涡旋光束传输40次后的各拓扑荷数的平均探测概率,右列表示相应拓扑荷数的平均探测概率和理论探测概率的对比。(a~b)大气折射率结构常数为Cn2=1×10-14 m-2/3;(c~d)大气折射率结构常数为Cn2=5×10-14 m-2/3;(e~f)大气折射率结构常数为Cn2=1×10-13 m-2/3
Figure 5. The topological charge of the vortex beam is 9, the average detection probability of each topological charge after 40 times of vortex beam transmission at different turbulence and the comparison between the average detection probability and the theoretical detection probability of the corresponding topological charge. (a~b) The refractive index structure constant of atmospheric turbulence is Cn2=1×10-14 m-2/3; (c~d) The refractive index structure constant of atmospheric turbulence is Cn2=5×10-14 m-2/3; (e~f) The refractive index structure constant of atmospheric turbulence is Cn2=1×10-13 m-2/3
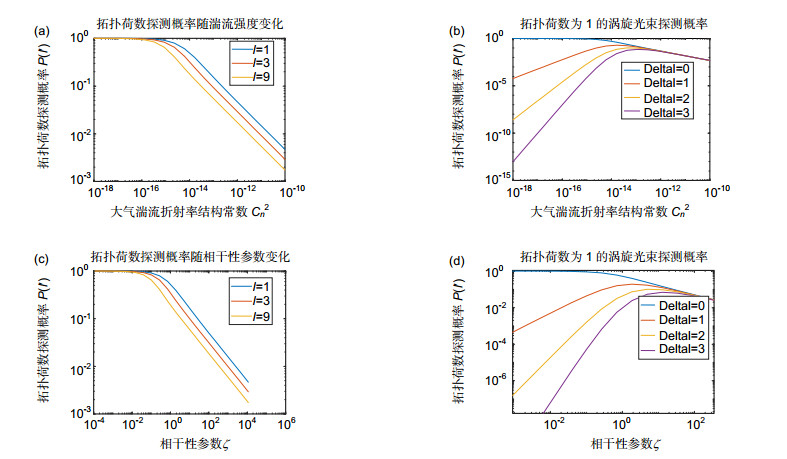
图 6 不同拓扑荷数的涡旋光束的探测概率随大气折射率结构常数(a)和相干性参数ζ (c)的变化;拓扑荷数为1的涡旋光束经过湍流大气后与邻近拓扑荷数的串扰情况随大气折射率结构常数(b)和相干性参数ζ (d)的变化
Figure 6. The detection probability of a vortex beam with different topological charge varies with the atmospheric refractive index structure constant (a) and with coherence parameter ζ (c); Crosstalk between vortex beams with original topological charge 1 and adjacent topological charges when propagating in different turbulent atmosphere (b) and with coherence parameter ζ (d)
-
[1] 柯熙政, 王姣.涡旋光束的产生、传输、检测及应用[M].北京:科学出版社, 2018.
Ke X Z, Wang J. Generation, transmission, detection and application of vortex beams[M]. Beijing: Science Press, 2018.
[2] Chen R S, Wang J H, Zhang X Q, et al. Fiber-based mode converter for generating optical vortex beams[J]. Opto-Electronic Advances, 2018, 1(7): 180003. http://d.old.wanfangdata.com.cn/Periodical/gdjz-e201807001
[3] Gibson G, Courtial J, Padgett M J, et al. Free-space information transfer using light beams carrying orbital angular momentum[J]. Optics Express, 2004, 12(22): 5448-5456. doi: 10.1364/OPEX.12.005448
[4] Wei S B, Wang D P, Lin J, et al. Demonstration of orbital angular momentum channel healing using a Fabry-Pérot cavity[J]. Opto-Electronic Advances, 2018, 1(5): 180006. http://d.old.wanfangdata.com.cn/Periodical/gdjz-e201805001
[5] 吕宏, 柯熙政.具轨道角动量光束用于光通信编码及解码研究[J].光学学报, 2009, 29(2): 331-335. http://d.old.wanfangdata.com.cn/Periodical/gxxb200902010
Lv H, Ke X Z. Research on the beam with orbital angular momentum used in encoding and decoding of optical communication[J]. Acta Optica Sinica, 2009, 29(2): 331-335. http://d.old.wanfangdata.com.cn/Periodical/gxxb200902010
[6] Yue Y, Bozinovic N, Ren Y X, et al. 1.6-Tbit/s muxing, transmission and demuxing through 1.1-km of vortex fiber carrying 2 OAM beams each with 10 wavelength channels[C]//Optical Fiber Communication Conference/National Fiber Optic Engineers Conference, 2013.
[7] Ren Y X, Huang H, Xie G D, et al. Atmospheric turbulence effects on the performance of a free space optical link employing orbital angular momentum multiplexing[J]. Optics Letters, 2013, 38(20): 4062-4065. doi: 10.1364/OL.38.004062
[8] Malik M, O'Sullivan M, Rodenburg B, et al. Influence of atmospheric turbulence on optical communications using orbital angular momentum for encoding[J]. Optics Express, 2012, 20(12): 13195-13200. doi: 10.1364/OE.20.013195
[9] 陈斐楠, 陈晶晶, 赵琦, 等.高阶贝塞尔高斯光束在非柯尔莫哥诺夫大气中的传输特性[J].中国激光, 2012, 39(9): 913001. http://d.old.wanfangdata.com.cn/Conference/8141015
Chen F N, Chen J J, Zhao Q, et al. Properties of high order bessel gaussian beam propagation in non-kolmogorov atmosphere turbulence[J]. Chinese Journal of Lasers, 2012, 39(9): 913001. http://d.old.wanfangdata.com.cn/Conference/8141015
[10] 江月松, 张新岗, 王帅会, 等.部分相干贝塞尔高斯光束在非柯尔莫哥诺夫湍流中的传输特性[J].光子学报, 2014, 43(1): 101001. http://d.old.wanfangdata.com.cn/Periodical/gzxb201401001
Jang Y S, Zhang X G, Wang S H, et al. Propagation of partially coherent bessel-gaussian beams in non-kolmogorov turbulence[J]. Acta Photonica Sinica, 2014, 43(1): 101001. http://d.old.wanfangdata.com.cn/Periodical/gzxb201401001
[11] 柯熙政, 王超珍.部分相干涡旋光束在大气湍流中传输时的光强分布[J].激光与光电子学进展, 2016, 53(11): 110604. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jgygdzxjz201611010
Ke X Z, Wang C Z. Intensity distribution of the partially coherent vortex beams propagating in atmospheric turbulence[J]. Laser & Optoelectronics Progress, 2016, 53(11): 110604. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jgygdzxjz201611010
[12] 柯熙政, 王超珍.部分相干离轴涡旋光束在大气湍流中的光强分布[J].光学学报, 2017, 37(1): 0101005. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=gxxb201701005
Ke X Z, Wang C Z. Intensity distribution of partially coherent off-axis vortex beam propagating in atmospheric turbulence[J]. Acta Optica Sinica, 2017, 37(1): 0101005. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=gxxb201701005
[13] Paterson C. Atmospheric turbulence and orbital angular momentum of single photons for optical communication[J]. Physical Review Letters, 2005, 94(15): 153901. doi: 10.1103/PhysRevLett.94.153901
[14] Anguita J A, Neifeld M A, Vasic B V. Turbulence-induced channel crosstalk in an orbital angular momentum-multiplexed free-space optical link[J]. Applied Optics, 2008, 47(13): 2414-2429. doi: 10.1364/AO.47.002414
[15] Tyler G A, Boyd R W. Influence of atmospheric turbulence on the propagation of quantum states of light carrying orbital angular momentum[J]. Optics Letters, 2009, 34(2): 142-144. doi: 10.1364/OL.34.000142
[16] Zhang Y X, Cang J. Effects of turbulent aberrations on probability distribution of orbital angular momentum for optical communication[J]. Chinese Physics Letters, 2009, 26(7): 074220. doi: 10.1088/0256-307X/26/7/074220
[17] 黎芳, 唐华, 江月松, 等.拉盖尔-高斯光束在湍流大气中的螺旋谱特性[J].物理学报, 2011, 60(1): 014204. http://d.old.wanfangdata.com.cn/Periodical/wlxb201101038
Li F, Tang H, Jiang Y S, et al. Spiral spectrum of laguerre-gaussian beams propagating in turbulent atmosphere[J]. Acta Physica Sinica, 2011, 60(1): 014204. http://d.old.wanfangdata.com.cn/Periodical/wlxb201101038
[18] Torner L, Torres J P, Carrasco S. Digital spiral imaging[J]. Optics Express, 2005, 13(3): 873-881. doi: 10.1364/OPEX.13.000873
[19] 黎芳.涡旋光束在中强度湍流大气中的传输特性[J].激光与光电子学进展, 2013, 50(7): 070101. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jgygdzxjz201307001
Li F. Propagation characteristics of optical vortices beam in intermediate fluctuation turbulent atmosphere[J]. Laser & Optoelectronics Progress, 2013, 50(7): 070101. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=jgygdzxjz201307001
[20] Cheng M J, Guo L X, Li J T, et al. Propagation of an optical vortex carried by a partially coherent laguerre-gaussian beam in turbulent ocean[J]. Applied Optics, 2016, 55(17): 4642-4648. doi: 10.1364/AO.55.004642
[21] Zhang W Y, Kuzyk M G. Effect of a thin optical Kerr medium on a laguerre-gaussian beam[J]. Applied Physics Letters, 2006, 89(10): 101103. doi: 10.1063/1.2345588
[22] 丁攀峰.整数与分数阶涡旋光束相位奇点的稳定性分析[J].华中科技大学学报(自然科学版), 2011, 39(5): 118-122.
Ding P F. Stabilization analysis of phase singularity of vortex beams with integral and fractional orders[J]. Journal of Huazhong University of Science and Technology (Natural Science Edition), 2011, 39(5): 118-122.
[23] He H, Friese M E J, Heckenberg N R, et al. Direct observation of transfer of angular momentum to absorptive particles from a laser beam with a phase singularity[J]. Physical Review Letters, 1995, 75(5): 826-829. doi: 10.1103/PhysRevLett.75.826
[24] Gradshteyn I S, Ryzhik I M. Table of Integrals, Series, and Products[M]. New York: Academic Press, 2014.
[25] 王立瑾, 李强, 魏宏刚, 等.大气湍流随机相位屏的数值模拟和验证[J].光电工程, 2007, 34(3): 1-4, 9. doi: 10.3969/j.issn.1003-501X.2007.03.001
Wang L J, Li Q, Wei H G, et al. Numerical simulation and validation of phase screen distorted by atmospheric turbulence[J]. Opto-Electronic Engineering, 2007, 34(3): 1-4, 9. doi: 10.3969/j.issn.1003-501X.2007.03.001
[26] 邹丽, 赵生妹, 王乐.大气湍流对轨道角动量态复用系统通信性能的影响[J].光子学报, 2014, 43(9): 0901001. http://d.old.wanfangdata.com.cn/Periodical/gzxb201409011
Zou L, Zhao S M, Wang L. The effects of atmospheric turbulence on orbital angular momentum-multiplexed system[J]. Acta Photonica Sinica, 2014, 43(9): 0901001. http://d.old.wanfangdata.com.cn/Periodical/gzxb201409011
[27] 王飞, 余佳益, 刘显龙, 等.部分相干光束经过湍流大气传输研究进展[J].物理学报, 2018, 67(18): 184203. doi: 10.7498/aps.67.20180877
Wang F, Yu J Y, Liu X L, et al. Research progress of partially coherent beams propagation in turbulent atmosphere[J]. Acta Physica Sinica, 2018, 67(18): 184203. doi: 10.7498/aps.67.20180877
[28] 彭浩.随机并行梯度下降波前控制算法研究[D].长沙: 国防科学技术大学, 2008.
Peng H. Research of the sotchastic parallel gradient descent algorithm for wavefront control technique[D]. Changsha: National University of Defense Technology, 2008.
[29] Lane R G, Glindemann A, Dainty J. Simulation of a Kolmogorov phase screen[J]. Waves in Random Media, 1992, 2(3): 209-224. doi: 10.1088/0959-7174/2/3/003
-


 E-mail Alert
E-mail Alert RSS
RSS

 下载:
下载: